quantitative ecology
Biological Diversity - the great
variety of life
Biological diversity can be
quantified in many different ways. The two main factors taken into account when
measuring diversity are richness and evenness. Richness is a measure of the
number of different kinds of organisms present in a particular area. For
example, species richness is the number of different species present. However,
diversity depends not only on richness, but also on evenness. Evenness compares
the similarity of the population size of each of the species present.
1. Richness
The number of species per sample is
a measure of richness. The more species present in a sample, the 'richer' the
sample. Species richness as a measure on its own takes no account of the number
of individuals of each species present. It gives as much weight to those
species which have very few individuals as to those which have many
individuals. Thus, one daisy has as much influence on the richness of an area
as 1000 buttercups.
2. Evenness
Evenness is a measure of the
relative abundance of the different species making up the richness of an area. To
give an example, we might have sampled two different fields for wildflowers.
The sample from the first field consists of 300 daisies, 335 dandelions and 365
buttercups. The sample from the second field comprises 20 daisies, 49
dandelions and 931 buttercups (see the table below). Both samples have the same
richness (3 species) and the same total number of individuals (1000). However,
the first sample has more evenness than the second. This is because the total number
of individuals in the sample is quite evenly distributed between the three
species. In the second sample, most of the individuals are buttercups, with
only a few daisies and dandelions present. Sample 2 is therefore considered to
be less diverse than sample 1.
|
Numbers of individuals
|
||
|
Flower Species
|
Sample 1
|
Sample 2
|
|
Daisy
|
300
|
20
|
|
Dandelion
|
335
|
49
|
|
Buttercup
|
365
|
931
|
|
Total
|
1000
|
1000
|
A community dominated by one or two
species is considered to be less diverse than one in which several different
species have a similar abundance.
As species richness and evenness increase, so
diversity increases. Simpson's Diversity Index is a measure of diversity which
takes into account both richness and evenness.
The term 'Simpson's Diversity Index' can actually
refer to any one of 3 closely related indices. Simpson's Index (D)
measures the probability that two individuals randomly selected from a sample
will belong to the same species (or some category other than species). There
are two versions of the formula for calculating D. Either is acceptable,
but be consistent.
The value of D ranges between 0 and
1
With this index, 0 represents infinite
diversity and 1, no diversity. That is, the bigger the value of D, the lower
the diversity. This is neither intuitive nor logical, so to get over this
problem, D is often subtracted from 1 to give:
Simpson's Index of
Diversity 1 - D
The value of this index also ranges
between 0 and 1, but now, the greater the value, the greater the sample
diversity. This makes more sense. In this case, the index represents the
probability that two individuals randomly selected from a sample will belong to
different species.
Another way of overcoming the problem of
the counter-intuitive nature of Simpson's Index is to take the reciprocal of
the Index:
Simpson's Reciprocal
Index 1 / D
The value of this index starts with 1 as
the lowest possible figure. This figure would represent a community containing
only one species. The higher the value, the greater the diversity. The maximum
value is the number of species (or other category being used) in the sample.
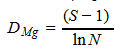


thank you for information rida, its so useful
BalasHapusKomentar ini telah dihapus oleh pengarang.
HapusTerimakasih kakak atas materinya
BalasHapusOverall, great riiid!
BalasHapusLebih baik lagi apabila diberi bullets atau numbering pada bagian yang belum diberi dan hanya diberi penebalan. Ohya, another suggestion is the title of this article needs to be more bigger to make it clearly seem :) semangat!! :)
terimakasih atas infonya, sudah lengkap dan disertai penjelasan serta rumus :)
BalasHapusNice information kakak
BalasHapus